Jack Wischmeyer
Inverse Trigonometric Functions
Inverse Sine Function
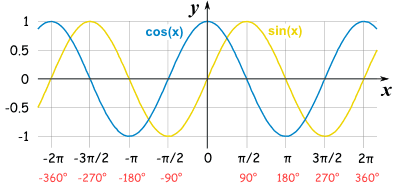
In order for an equation to be a function, we know that it must pass the Horizontal Line Test. The three basic trigonometric functions that do not pass this test are the sine, cosine, and tangent functions.
As we can clearly see, this graph does not pass the horizontal line test within the range of the function. However, in order to effectively use any of the three functions sine, cosine, and tangent, we must "change" the rules of the function. By restricting the domain of the function to (-3.14/2,3.14/2), the function can possess the properties of holding it's whole range, and having the function pass the horizontal line test.
Using the restricted domain function mentioned above, we can develop a new set of equations:
y=arcsinx
Each of these functions are the inverse of it's counterpart.
y=arccosx
y=arctanx
Below is a useful table discussing the properties of the trigonometric functions:
Function Domain Range
y=arcsinx if and only if sin y=x (-1,1) (-3.14/2,3.14/2)
y=arccosx if and only if cos y=x (-1,1) (0,3.14)
y=arctanx if and only if tan y=x All Real Numbers (-3.14/2,3.14/2)
As we learned earlier how the composite of a function and it's inverse equals x, the same properties are applied here. If the domain of the trigonometric functions is (-1,1) and the range is (-3.14/2,3.14/2), then the following is true:
sin(arcsinx)=x arcsin(sinx)=x
cos(arccosx)=x arcos(cosx)=x
tan(arctanx)=x arctan(tanx)=x
Ultimately, the basic properties of the trigonometric functions are not that different from what we have seen before.